目录
- 1 TreeMap基本介绍
- 2 红黑树数据结构回顾
- 3 成员变量
- 4 内部类Entry
- 5 构造函数
-
6 重要方法分析
- 6.1 get方法分析
- 6.2 put方法分析
- 6.3 插入调整函数fixAfterInsertion()解析
- 6.4 删除方法remove()解析
- 6.5 删除调整函数fixAfterDeletion()解析
- 6.6 寻找后继函数successor()解析
-
7 解惑:
-
- 1 TreeMap支持key自定义排序,而红黑树对key的固定的排序规则,两者如何兼容的?
-
1 TreeMap基本介绍
- Java TreeMap实现了SortedMap接口,也就是说会按照key的大小顺序对Map中的元素进行排序
- key大小的评判可以通过其本身的自然顺序(natural ordering),也可以通过构造时传入的比较器(Comparator)。
- TreeMap底层通过红黑树实现
- TreeMap是非同步的。可以通过如下方式将TreeMap包装成同步的:SortedMap m = Collections.synchronizedSortedMap(new TreeMap(...));
- TreeMap 跟 HashMap是两种不同的结构,TreeMap没有使用hash相关概念
2 红黑树数据结构回顾
- 每个节点颜色不是黑色,就是红色
- 根节点是黑色的
- 红色节点不能连续
- 对于每个节点,从该节点到其树尾端的简单路径上,均包含相同数目的黑色节点
3 成员变量
private final Comparator<? super K> comparator; //排序器,如果空,按照key的字典顺序来排序(升序);comparator为空时用Comparable的排序接口
private transient Entry<K,V> root; //根节点
private transient int size = 0; //树中entry个数 ,即红黑树大小
private transient int modCount = 0; //数结构被修改的次数的
/**
* Fields initialized to contain an instance of the entry set view
* the first time this view is requested. Views are stateless, so
* there's no reason to create more than one.
*/
private transient EntrySet entrySet;
private transient KeySet<K> navigableKeySet;
private transient NavigableMap<K,V> descendingMap;
/**
* Dummy value serving as unmatchable fence key for unbounded
* SubMapIterators
*/
private static final Object UNBOUNDED = new Object();
private static final boolean RED = false; //红节点 默认false
private static final boolean BLACK = true; // 黑节点 默认true
4 内部类Entry
它是组成树的节点的类型
static final class Entry<K,V> implements Map.Entry<K,V> {
K key; // key
V value; //value
Entry<K,V> left; //左孩子
Entry<K,V> right; //右孩子
Entry<K,V> parent; //父节点
boolean color = BLACK; //默认黑色
//根据 key value 父节点创建新节点
Entry(K key, V value, Entry<K,V> parent) {
this.key = key;
this.value = value;
this.parent = parent;
}
public K getKey() {
return key;
}
public V getValue() {
return value;
}
//替换value,返回旧value
public V setValue(V value) {
V oldValue = this.value;
this.value = value;
return oldValue;
}
// 重写equals方法:key 和 value的引用都相等
public boolean equals(Object o) {
if (!(o instanceof Map.Entry))
return false;
Map.Entry<?,?> e = (Map.Entry<?,?>)o;
return valEquals(key,e.getKey()) && valEquals(value,e.getValue());
}
//重写hashCode方法,返回 key 和 value的hashCode 的异或运算结构
public int hashCode() {
int keyHash = (key==null ? 0 : key.hashCode());
int valueHash = (value==null ? 0 : value.hashCode());
return keyHash ^ valueHash;
}
public String toString() {
return key + "=" + value;
}
}
5 构造函数
// 构造函数一:不指定排序器。按照key的字典顺序来排序(升序)
public TreeMap() {
comparator = null;
}
// 构造函数二:指定排序器
public TreeMap(Comparator<? super K> comparator) {
this.comparator = comparator;
}
//构造函数三:构造并返回跟参数m有相同键值映射结构的treeMap(m变为红黑树)
public TreeMap(Map<? extends K, ? extends V> m) {
comparator = null;
putAll(m);
}
//构造函数四:构造并返回跟参数m(有序的)有相同键值映射结构的treeMap
public TreeMap(SortedMap<K, ? extends V> m) {
comparator = m.comparator();
try {
buildFromSorted(m.size(), m.entrySet().iterator(), null, null);
} catch (java.io.IOException cannotHappen) {
} catch (ClassNotFoundException cannotHappen) {
}
}
Comparator Integer类型倒序排序
public static void main(String[] args) {
TreeMap treeMap = new TreeMap<Integer ,String>(new ComparatorObj());
treeMap.put(2,"ss");
treeMap.put(3,"sss");
System.out.println(treeMap);
}
static class ComparatorObj implements Comparator<Integer>{
@Override
public int compare(Integer o1, Integer o2) {
return o2-o1; //倒序排序
}
}
输出结果:
2022-07-11 18:02:23,871 [INFO] main: {3=sss, 2=ss}
6 重要方法分析
6.1 get方法分析
(实际调用getEntry(Object key))
- get(Object key)方法是对接口Map的方法实现
- get(Object key)方法转为对getEntry(Object key)方法的实现分析:算法思想是根据key的自然顺序(或者比较器顺序)对二叉查找树进行查找,直到找到满足k.compareTo(p.key) == 0的entry,再返回entry的value。
源码分析如下:
public V get(Object key) {
Entry<K,V> p = getEntry(key); //根据key找到entry,再返回其value
return (p==null ? null : p.value);
}
//重点分析该方法
final Entry<K,V> getEntry(Object key) {
if (comparator != null)
return getEntryUsingComparator(key);
if (key == null)
throw new NullPointerException(); //key非空校验
@SuppressWarnings("unchecked")
Comparable<? super K> k = (Comparable<? super K>) key; //自然顺序,使用Comparable的排序接口
Entry<K,V> p = root;
while (p != null) { //从根节点开始 循环遍历
int cmp = k.compareTo(p.key); //compareTo:左边减去右边
if (cmp < 0) //参数key值小于父节点key
p = p.left; //取左子节点
else if (cmp > 0)
p = p.right; //参数key值大于父节点key,取右子节点
else
return p; //key相等,则直接返回当前entry
}
return null;
}
查询方法说明:
- 在while循环外,创建动态游标节点,游标首次指向root节点,以游标!=null作为循环条件
- 在while循环内,根据compareTo结果,取游标的左子节点或右子节点,作为新的游标
- 找到满足k.compareTo(p.key) == 0的entry,退出循环
getEntryUsingComparator源码:
//提供自定义排序器的查询找方法,原理类似
final Entry<K,V> getEntryUsingComparator(Object key) {
@SuppressWarnings("unchecked")
K k = (K) key;
Comparator<? super K> cpr = comparator;
if (cpr != null) {
Entry<K,V> p = root;
while (p != null) {
int cmp = cpr.compare(k, p.key); //cpr.compare 第一个参数减去第二个参数
if (cmp < 0)
p = p.left;
else if (cmp > 0)
p = p.right;
else
return p;
}
}
return null;
}
6.2 put方法分析
public V put(K key, V value) {
Entry<K,V> t = root;
// 情况一:根节点为空,将当前key value作为root
if (t == null) {
compare(key, key); //key为null则抛异常
root = new Entry<>(key, value, null);//初始化root
size = 1; //叶子个数+1
modCount++; // 结构修改次数自增
return null; //新叶子,所以old value 为空
}
// 情况二:如果找到key相同的,则更新value ,过程类似get方法
int cmp;
Entry<K,V> parent;
Comparator<? super K> cpr = comparator;
if (cpr != null) {
do {
parent = t;
cmp = cpr.compare(key, t.key);
if (cmp < 0)
t = t.left;
else if (cmp > 0)
t = t.right;
else
return t.setValue(value);
} while (t != null);
}
else {
if (key == null)
throw new NullPointerException();
@SuppressWarnings("unchecked")
Comparable<? super K> k = (Comparable<? super K>) key;
do {
parent = t;
cmp = k.compareTo(t.key);
if (cmp < 0)
t = t.left;
else if (cmp > 0)
t = t.right;
else
return t.setValue(value);
} while (t != null);
}
//情况三:没有相同的key,则添加新叶子。
//经过上面的两种遍历,完成了二分法查找,找到适合插入的地方:parent。
Entry<K,V> e = new Entry<>(key, value, parent); //创建新的entry
//确定新增叶子作为parent的左孩子还是右孩子,插入的动作完成
if (cmp < 0)
parent.left = e;
else
parent.right = e;
fixAfterInsertion(e); //插入完成后,对二叉树进行调整
size++;
modCount++;
return null;
}
//这个方法实际上是对key做null检查,如果是null会抛出异常(测试代码验证过)
public TreeMap(Comparator<? super K> comparator) {
this.comparator = comparator;
}
put方法说明:
- 如果root为空,则新增的entry作为root
- 遍历搜索是否存在相同的key,存在则替换value。这过程也是二叉排序树的二分查找法:找到了作为插入点的parent。
- 插入操作:找到parent,并将其left或者right指向新的entry。
- 如果是插入,则需要对红黑树进行结构调整。 (插入:节点默认为红色,root节点:设置为黑色,覆盖节点:颜色保持不变)
- 维护成员变量:size,modCount。
6.3 插入调整函数fixAfterInsertion()解析
/** From CLR */
private void fixAfterInsertion(Entry<K,V> x) {
x.color = RED; //新增节点默认为红色,再进行规则判断
// 从树末端开始遍历:父节点是红色,则需要对树进行调整
while (x != null && x != root && x.parent.color == RED) {
if (parentOf(x) == leftOf(parentOf(parentOf(x)))) {
Entry<K,V> y = rightOf(parentOf(parentOf(x)));
if (colorOf(y) == RED) {
setColor(parentOf(x), BLACK);
setColor(y, BLACK);
setColor(parentOf(parentOf(x)), RED);
x = parentOf(parentOf(x));
} else {
if (x == rightOf(parentOf(x))) {
x = parentOf(x);
rotateLeft(x);
}
setColor(parentOf(x), BLACK);
setColor(parentOf(parentOf(x)), RED);
rotateRight(parentOf(parentOf(x)));
}
} else {
Entry<K,V> y = leftOf(parentOf(parentOf(x)));
if (colorOf(y) == RED) {
setColor(parentOf(x), BLACK);
setColor(y, BLACK);
setColor(parentOf(parentOf(x)), RED);
x = parentOf(parentOf(x));
} else {
if (x == leftOf(parentOf(x))) {
x = parentOf(x);
rotateRight(x);
}
setColor(parentOf(x), BLACK);
setColor(parentOf(parentOf(x)), RED);
rotateLeft(parentOf(parentOf(x)));
}
}
}
root.color = BLACK; //在遍历外面,确保root一定是黑色
}
方法说明:
- 新增的节点默认为红色,并从树末端往上遍历
- 如果新增节点的父亲是红色,则需要进行结构调整
- 结构调整这部分有点复杂,回头再深入理解todo
6.4 删除方法remove()解析
知识回顾:
二叉排序树的删除过程:(情况一,treeMap用后继代替,其实用前驱或者后继是一样的)
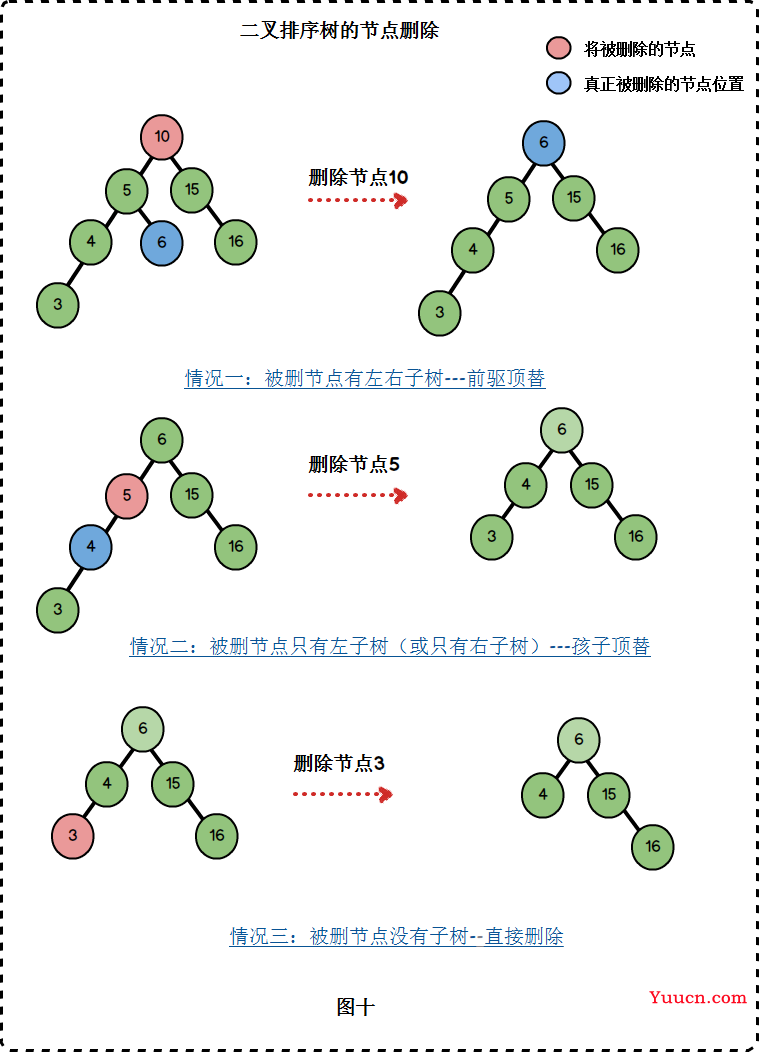
源码如下:
// 调用getEntry(key)找到对应entry,调用deleteEntry 删除节点
public V remove(Object key) {
Entry<K,V> p = getEntry(key);
if (p == null)
return null;
V oldValue = p.value;
deleteEntry(p);
return oldValue;
}
//执行删除操作
private void deleteEntry(Entry<K,V> p) {
//先对全局变量modCount、size 进行调整
modCount++;
size--;
//情况1:左右孩子都不为空:后继节点代替
if (p.left != null && p.right != null) {
Entry<K,V> s = successor(p); //寻找后继 (另外分析)
//将删除点的key、value、引用分别更新为代替节点
p.key = s.key;
p.value = s.value;
p = s; //
}
//情况2:有1个孩子
Entry<K,V> replacement = (p.left != null ? p.left : p.right);
if (replacement != null) {
replacement.parent = p.parent; //左孩子父亲更新删除节点的父亲
//父亲为root,则后继变为新的root
if (p.parent == null)
root = replacement;
//左孩子顶上
else if (p == p.parent.left)
p.parent.left = replacement;
//右孩子顶上
else
p.parent.right = replacement;
// 删除节点的left、right、parent置空:被移除出树
p.left = p.right = p.parent = null;
// 删除黑色节点:调整结构
if (p.color == BLACK)
fixAfterDeletion(replacement);
} else if (p.parent == null) { //删除root节点
root = null;
} else { // 情况1:没孩子
if (p.color == BLACK)
fixAfterDeletion(p);
//将父亲的左孩子或者有孩子清空
if (p.parent != null) {
if (p == p.parent.left)
p.parent.left = null;
else if (p == p.parent.right)
p.parent.right = null;
p.parent = null;
}
}
}
删除说明:
- 删除过程遵从二叉排序树的删除特点(1、有一个孩子则孩子顶上;2两个孩子就用后继顶上,没有孩子则直接移除)
- 节点删除,即left、right、parent置空;删除后,需要更新父亲节点的的左孩子或右孩子
- 考虑是否需要更新全局变量root节点
- 只有删除点是BLACK的时候,才会触发调整函数,因为删除RED节点不会破坏红黑树的任何约束,而删除BLACK节点会破坏规则4。
6.5 删除调整函数fixAfterDeletion()解析
private void fixAfterDeletion(Entry<K,V> x) {
while (x != root && colorOf(x) == BLACK) {
if (x == leftOf(parentOf(x))) {
Entry<K,V> sib = rightOf(parentOf(x));
if (colorOf(sib) == RED) {
setColor(sib, BLACK);
setColor(parentOf(x), RED);
rotateLeft(parentOf(x));
sib = rightOf(parentOf(x));
}
if (colorOf(leftOf(sib)) == BLACK &&
colorOf(rightOf(sib)) == BLACK) {
setColor(sib, RED);
x = parentOf(x);
} else {
if (colorOf(rightOf(sib)) == BLACK) {
setColor(leftOf(sib), BLACK);
setColor(sib, RED);
rotateRight(sib);
sib = rightOf(parentOf(x));
}
setColor(sib, colorOf(parentOf(x)));
setColor(parentOf(x), BLACK);
setColor(rightOf(sib), BLACK);
rotateLeft(parentOf(x));
x = root;
}
} else { // symmetric
Entry<K,V> sib = leftOf(parentOf(x));
if (colorOf(sib) == RED) {
setColor(sib, BLACK);
setColor(parentOf(x), RED);
rotateRight(parentOf(x));
sib = leftOf(parentOf(x));
}
if (colorOf(rightOf(sib)) == BLACK &&
colorOf(leftOf(sib)) == BLACK) {
setColor(sib, RED);
x = parentOf(x);
} else {
if (colorOf(leftOf(sib)) == BLACK) {
setColor(rightOf(sib), BLACK);
setColor(sib, RED);
rotateLeft(sib);
sib = leftOf(parentOf(x));
}
setColor(sib, colorOf(parentOf(x)));
setColor(parentOf(x), BLACK);
setColor(leftOf(sib), BLACK);
rotateRight(parentOf(x));
x = root;
}
}
}
setColor(x, BLACK);
}
说明:结构调整这部分有点复杂,回头再深入理解todo
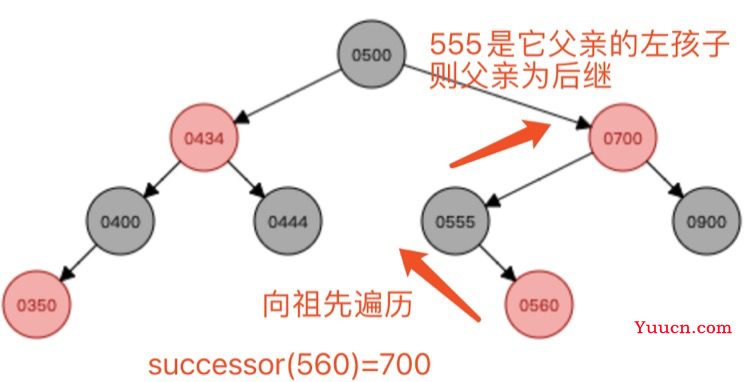
6.6 寻找后继函数successor()解析
//寻找任意节点后继
static <K,V> TreeMap.Entry<K,V> successor(Entry<K,V> t) {
if (t == null)
return null;
//情况1:右孩子不为空,向后代遍历:找到右孩子中孙子最小的那个节点(不断寻找left,直至为空)
else if (t.right != null) {
Entry<K,V> p = t.right;
while (p.left != null)
p = p.left;
return p;
} else {
// 情况2:右孩子为空,向祖先遍历,当任意节点是它父亲的左孩子时,则该节点的父亲为t的后继
Entry<K,V> p = t.parent;
Entry<K,V> ch = t;
while (p != null && ch == p.right) {
ch = p;
p = p.parent;
}
return p;
}
}
寻找后继的算法说明:
对于任意k:
- 情况一:k的右孩子不为空,向后代遍历:找到右孩子的子孙子中辈分最低的左孩子(不断寻找left,直至为空)
- 情况二:key的右孩子为空,向祖先遍历:当任意节点是它父亲的左孩子时,则该节点的父亲为t的后继
右孩子不为空的后继寻找:
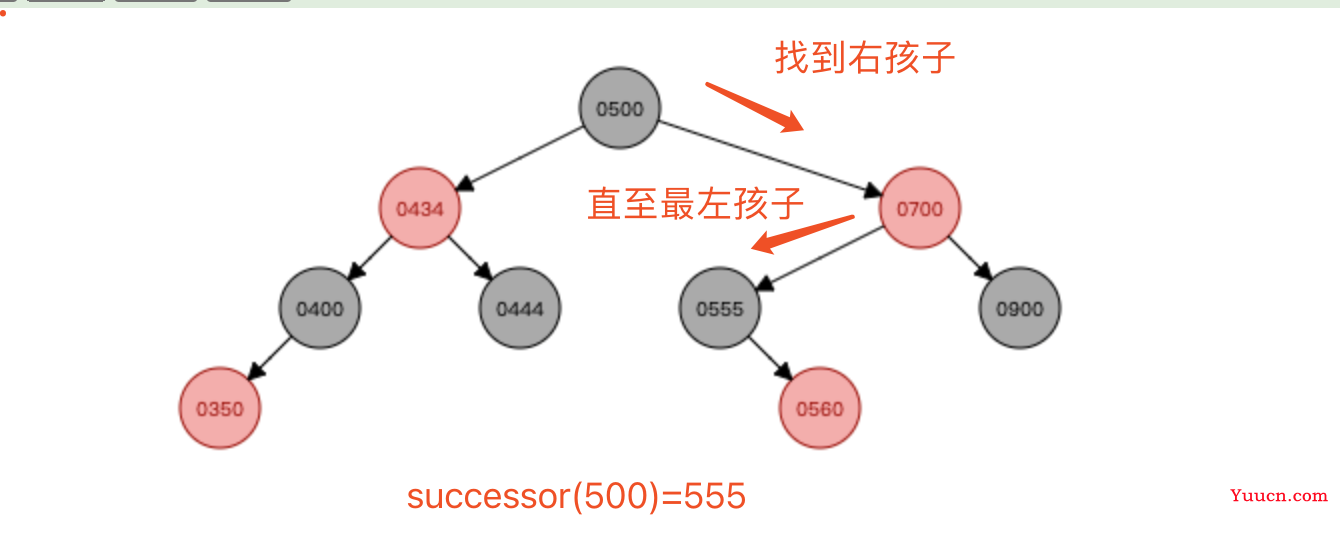
右孩子为空为空的后继寻找:
7 解惑:
1 TreeMap支持key自定义排序,而红黑树对key的固定的排序规则,两者如何兼容的?
- 支持key自定义排序:指通过自定义的排序器,计算出任意key相对其它key的大小关系
- 红黑树对key的固定的排序:指按照红黑树的数据结构(二叉排序树+红黑节点规则),来组织key的树状结构,其中二叉排序的大小关系是根据排序器的计算出来的
- 两者不冲突